The Euler-Poincaré Formula
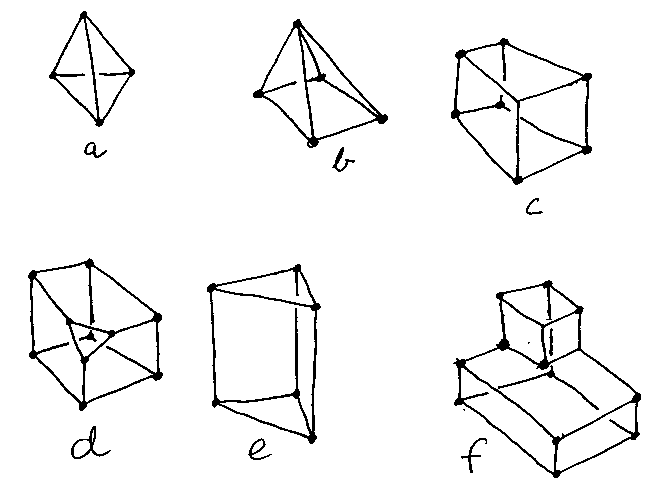
Compile a table of the number of faces, F, edges, E, and vertices, V, of the polyhedra a....f in the figure below. Leave two rows at the bottom of your table; you're going to add more polyhedra to it in a moment.

Can you devise a formula relating F, E, and V that is true for all the shapes in your table?
Can you prove that your formula is true for all polyhedra? (Hint: Imagine two simple polyhedra for which you know that the formula is true because you've counted; imagine also that each has one face that is identical to a face on the other one; what happens if you glue those two faces together to make one bigger more complicated polyhedron?)
What about holes (polyhedra g and h)? For polyhedron g think about your proof: what would happen with two simple polyhedra that each just happened to have two faces that matched two on the other, so that when you glued them together they made a doughnut (or torus)?
Shape h is a bit harder: you have to count rings in faces that are flat but which have holes punched through them.

Suppose the top and bottom edges of shape e were curved so that it was a cylinder. Would that make any difference? What about a real cylinder, a cone, and a sphere?
© Adrian Bowyer 1996