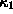 and
and 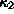 .
The Gaussian curvature equals the product of the principal curvatures.
The Mean curvature equals the arithmetic average of the principal
curvatures.
.
The Gaussian curvature equals the product of the principal curvatures.
The Mean curvature equals the arithmetic average of the principal
curvatures.



Gaussian (K) and Mean (H) curvatures are the most widely used
indicators for surface shape classification in range image analysis. The HK
segmentation[1,2,4] was introduced by Besl
in 1986. He defines Gaussian and Mean curvatures, which are calculated
from the two principal curvatures 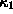 and
and 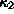 .
The Gaussian curvature equals the product of the principal curvatures.
The Mean curvature equals the arithmetic average of the principal
curvatures.
.
The Gaussian curvature equals the product of the principal curvatures.
The Mean curvature equals the arithmetic average of the principal
curvatures.

Image points can be labelled as belonging to a viewpoint- independent surface shape class type based on the combination of the signs from the Gaussian and Mean curvatures as shown in Table 1.
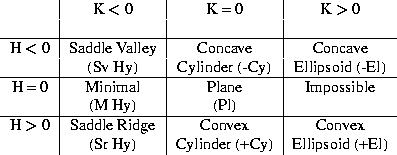
Table 1: Classification for the HK segmentation based on the
sign H and K
Koenderink defined an alternative curvature representation[3]. His
approach (SC classification) decouples the shape and the magnitude
of the curvedness. The surface in terms of relative curvature remains
invariant under changes in scale. He defined a shape index S, which
is a number in the range [-1,1]. The index covers all shapes except
for the planar shape which has an indeterminate shape index
(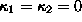 ). The shape index provides a continuous
gradation between shapes, such as concave shapes (-1 < S < -1/2),
hyperboloid shapes (-1/2 < S < 1/2) and convex shapes (1/2 < S < 1).
The image points can be classified as shown
in Table 2. We use the positive principal curvatures
(
). The shape index provides a continuous
gradation between shapes, such as concave shapes (-1 < S < -1/2),
hyperboloid shapes (-1/2 < S < 1/2) and convex shapes (1/2 < S < 1).
The image points can be classified as shown
in Table 2. We use the positive principal curvatures
(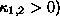 for convex objects.
Beside the shape index, Koenderink introduced the positive value C for
describing the magnitude of the curvedness at a point. It is a
measure of how highly or gently curved a point is. At a point
that has no curvedness the value becomes zero.
Therefore, this variable can be used to recognise a plane surface.
for convex objects.
Beside the shape index, Koenderink introduced the positive value C for
describing the magnitude of the curvedness at a point. It is a
measure of how highly or gently curved a point is. At a point
that has no curvedness the value becomes zero.
Therefore, this variable can be used to recognise a plane surface.


Table 2: Classification for Koenderink's approach based on the
shape index (S)


