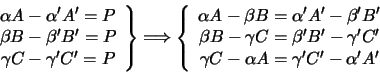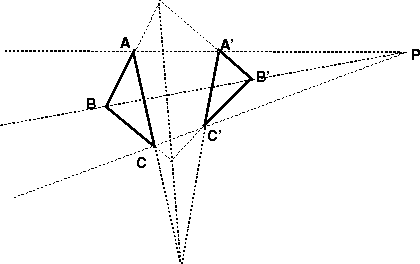
Desargues Theorem

Projective geometry was invented by the French mathematician Desargues (1591-1661) (for a biography in French, see http://bib1.ulb.ac.be/coursmath/bio/desargue.htm). One of his theorems is considered to be a cornerstone of the formalism. It states that ``Two triangles are in perspective from a point if and only if they are in perspective from a line'' (see fig. 2.1):

Theorem: Let A, B, C and A', B, C' be two triangles in the (projective) plane. The lines AA', BB', CC' intersect in a single point if and only if the intersections of corresponding sides (AB, A'B'), (BC, B'C'), (CA, C'A') lie on a single line.

  

Figure 2.1: Two triangles in a Desargueian configuration

The theorem has a clear self duality: given two triplets of lines $\{a, b, c\}$ and $\{a', b', c'\}$ defining two triangles, the intersections of the corresponding sides lie on a line if and only if the lines of intersection of the corresponding vertices intersect in a point.

We will give an algebraic proof: Let P be the common intersection of AA', BB', CC'. Hence there are scalars $\alpha,\beta,\gamma,\alpha',\beta',\gamma'$ such that:

$$\left. \begin{array}{c} \alpha A - \alpha' A' = P\\ \beta B... ...mma C - \alpha A = \gamma' C' - \alpha' A' \end{array}\right.$$

This indicates that the point $\alpha A - \beta B$ on the line AB also lies at $\alpha' A' - \beta' B'$ on the line A'B', and hence corresponds to the intersection of AB and A'B', and similarly for $\beta B-\gamma C=BC\cap B'C'$ and $\gamma C - \alpha A=CA\cap C'A'$. But given that

$$(\alpha A - \beta B) +(\beta B - \gamma C) +(\gamma C - \alpha A) =0$$

the three intersection points are linearly dependent, i.e. collinear. $\sqcup \!\!\!\! \sqcap$

Exercise 2.4   :  The sun (viewed as a point light source) casts on the planar ground the shadow A'B'C' of a triangular roof ABC (see fig. 2.2). Consider a perspective image of all this, and show that it is a Desargueian configuration. To which 3D line does the line of intersections in Desargues theorem correspond? If a further point D in the plane ABC produces a shadow D', show that it is possible to reconstruct the image of D from that of D'.

  

Figure 2.2: Shadow of a triangle on a planar ground